
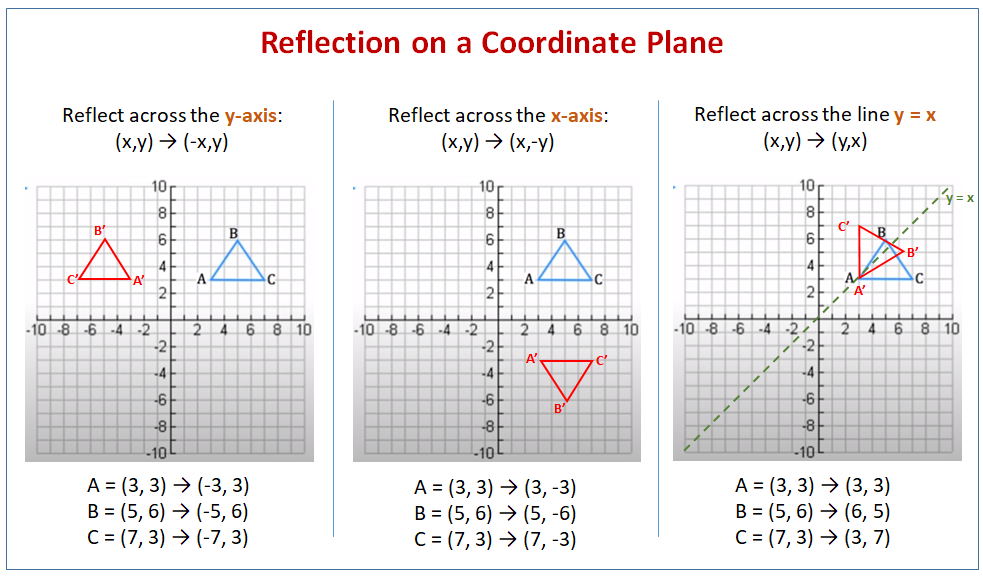
The axis of symmetry is simply the vertical line that we are performing the reflection across.
Coordinate rule for reflection over y axis how to#
But before we go into how to solve this, it's important to know what we mean by "axis of symmetry". In some cases, you will be asked to perform vertical reflections across an axis of symmetry that isn't the y-axis.
Step 3: Divide these points by (-1) and plot the new pointsįor a visual tool to help you with your practice, and to check your answers, check out this fantastic link here. Step 2: Identify easy-to-determine points Step 1: Know that we're reflecting across the y-axis Below are several images to help you visualize how to solve this problem. Don't pick points where you need to estimate values, as this makes the problem unnecessarily hard. When we say "easy-to-determine points" what this refers to is just points for which you know the x and y values exactly. Remember, the only step we have to do before plotting the f(-x) reflection is simply divide the x-coordinates of easy-to-determine points on our graph above by (-1). Given the graph of y = f ( x ) y=f(x) y = f ( x ) as shown, sketch y = f ( − x ) y = f(-x) y = f ( − x ). The best way to practice drawing reflections over y axis is to do an example problem: In order to do this, the process is extremely simple: For any function, no matter how complicated it is, simply pick out easy-to-determine coordinates, divide the x-coordinate by (-1), and then re-plot those coordinates.

One of the most basic transformations you can make with simple functions is to reflect it across the y-axis or another vertical axis.

Before we get into reflections across the y axis, make sure you've refreshed your memory on how to do simple vertical translation and horizontal translation.


 0 kommentar(er)
0 kommentar(er)
